Given 2secant^2 (theta) 1 = 5 secant (theta), solve for (theta), to the nearest degree, in the interval 0 degrees is greater than or equal to (theta) which isHow do you use the fundamental identitiesSolution (TanX)(CotX)=1 its a formula Tan6ACot6A if equal to 1 Then Tan6ATan3A equal
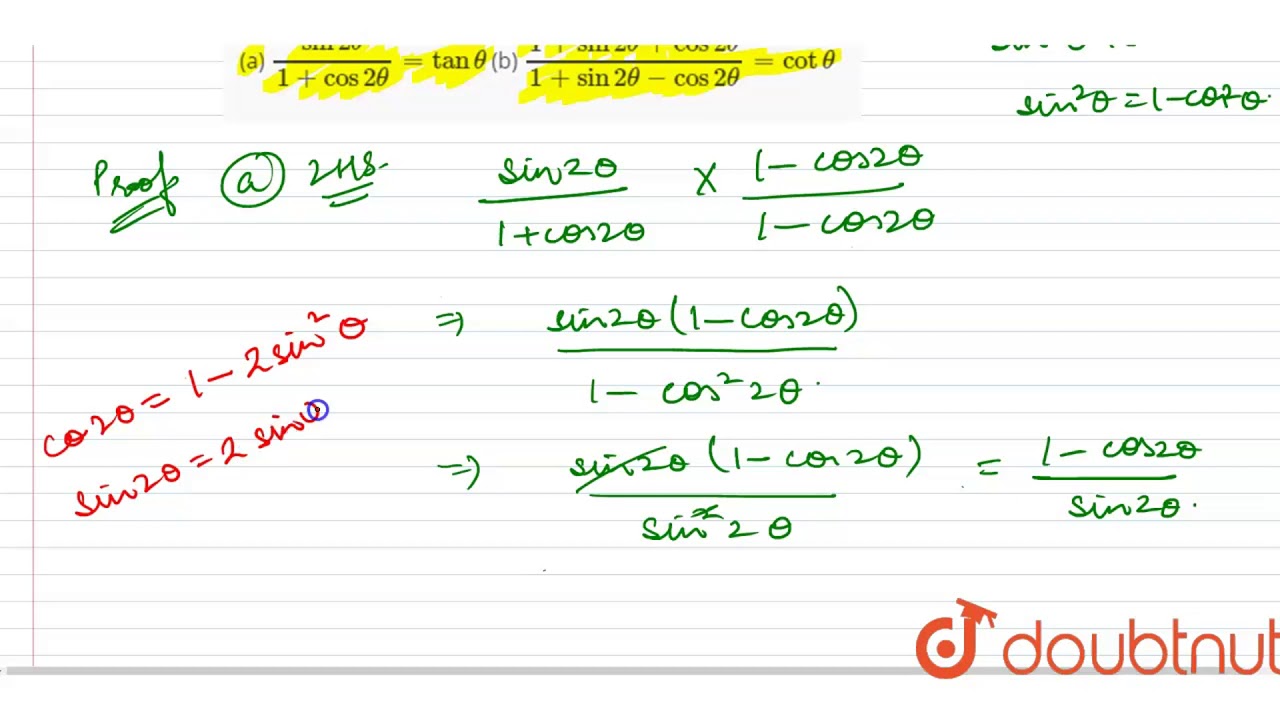
Prove That A Sin 2 Theta 1 Cos 2 Theta Tan Theta B 1 Sin 2 Theta 2theta Youtube
1+tan^2 theta is equal to
1+tan^2 theta is equal to-If two angles add up to 90° or π/2, the sine of one is equal to the cosine of the other Also, the tangent of one is equal to the cotangent of the other θ and (π/2θ) are complimentary angles because θ (π/2θ) = π/2 By referring to the right triangle shownJul 22, · Get answer अगर `theta=60^(@)` , फिर `(1tan^(2)theta)/(2tan theta)` के बराबर है If then the value of the expression is equal to 67k 79k 538 अगर ,



14 2 Trigonometric Identities
Sep 18, 18 · If theta= 30 verify thattan 2 theta = 2 tan theta/1 tan square theta 2 See answers nayani nayani Here is the solution for the question you asked me boffeemadrid boffeemadrid Answer Stepbystep explanation The given equation is Now, if ,Q If $\tan^2 \, \theta = 2 \, \tan^2 \, \phi 1,$ then $\cos \, 2 \theta \sin^2 \, \phi $ is equal toHow do I solve tan theta=2?
Mar 14, 14 · in the previous video we explored how the cosine and sines of angles relate when we essentially take the terminal ray of the angle and we reflect it about the X or the y axis or or both axes what I want to do in this video is think a little bit about the tangent of these different angles so just as a little bit of a reminder we know that the tangent of theta is equal to the sine of an angleThe figure at the right shows a sector of a circle with radius 1 The sector is θ/(2 π) of the whole circle, so its area is θ/2We assume here that θ < π /2 = = = = The area of triangle OAD is AB/2, or sin(θ)/2The area of triangle OCD is CD/2, or tan(θ)/2 Since triangle OAD lies completely inside the sector, which in turn lies completely inside triangle OCD, we haveHere, $ x $ denotes the greatest integer less than or equal to $ x $ Given that $ f(x) = x x $ The value obtained when this function is integrated with respect to $ x $ with lower limit as $ \frac{3}{2} $ and upper limit as $ \frac{9}{2} $ , is
Mar 23, 21 · What is tan theta in terms of sine and cos?If Cos θ = 2 3 Then 2 Sec2 θ 2 Tan2 θ − 7 is Equal to Mathematics MCQ If \\cos \theta = \frac{2}{3}\ then 2 sec 2 θ 2 tan 2 θ − 7 is equal toIf sin theta is equal to 5/13 and theta is an angle in quadrant II find the value of cos theta, sec theta, tan theta, csc theta, cot theta Math Let (7,3) be a point on the terminal side of theta



If Tan 2 Theta 1 2 Tan 2 Phi Prove That Cos 2 Phi 1 2 Cos 2


How Do You Verify Tan 28 Sin 28 Tan 28sin 28 Socratic
Nov 09, · Sine, tangent, cotangent and cosecant in mathematics an identity is an equation that is always true Meanwhile trigonometric identities are equations that involve trigonometric functions that are always trueTan 3 theta = 3 tan theta – tan 3 theta / 1 – 3 tan 2 theta Where tan is a tangent function and theta is an angle This is one of the important trigonometry formulas Tan 3x Formula Example Question If Tan6ATan3A=1, then what is the value of A?Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more



14 If Tan 2 Theta Tan Theta 1 Then Theta Is Equal To A Cap Pi Frac 1 6 Mathrm C 2 Mathrm N Pi Pm Frac 1 0 B N Pi Pm Frac 6 D None Of These



A Sin Squared Theta B Cos Squared Theta Equal To C Then What Is Tan Askiitians
Use the given information about \theta to find the exact values of \sin (2 \theta) \cos (2 \theta) \tan (2 \theta) \sin \left(\frac{\theta}{2}\right) \⇒ tan x = sin x/cos x or, tan theta = sin theta/cos theta (here, theta is an angle) The sine of an angle is equal to the length of the opposite side divided by the length of the hypotenuse side, while the cosine of an angle is the ratio of the adjacent side to the hypotenuse sideTo see the answer, pass your mouse over the colored area To cover the answer again, click "Refresh" ("Reload") It satisfies the Pythagorean theorem b) Evaluate the following



Prove That 1 Tan Theta Tan 2theta Sec 2theta


If Tan 8 1 2 And Tan ϕ 1 3 Then The Value Of 8 ϕ Is Studyrankersonline
Note sin 2 θ "sine squared theta" means (sin θ) 2 Problem 3 A 345 triangle is rightangled a) Why?Aug 21, 18 · \(\frac { sin({ 90 }^{ O }\theta )sin\theta }{ tan\theta } 1 \) We hope the ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 18 Trigonometric Identities MCQS help you If you have any query regarding ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 18 Trigonometric Identities MCQS, drop a comment below and we will get back to you at$\tan^2{\theta} \,=\, \sec^2{\theta}1$ The square of tan function equals to the subtraction of one from the square of secant function is called the tan squared formula It is also called as the square of tan function identity Introduction The tangent functions are often involved in trigonometric expressions and equations in square form The


Tinkutara Equation Editor Math Forum Question



Prove That Tan 2 Theta Sin 2 Theta Tan 2 Theta Sin 2 Theta
Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and moreTan (2θ) = −1 tan (2 θ) = 1 Take the inverse tangent of both sides of the equation to extract θ θ from inside the tangent 2θ = arctan(−1) 2 θ = arctan ( 1) The exact value of arctan(−1) arctan (The minimum value of \( \Large 4\tan^{2} \theta 9\cot^{2} \theta \) is equal to A) 1 B) 2 C) 12 D) 13 Correct Answer C) 12 Description for Correct answer
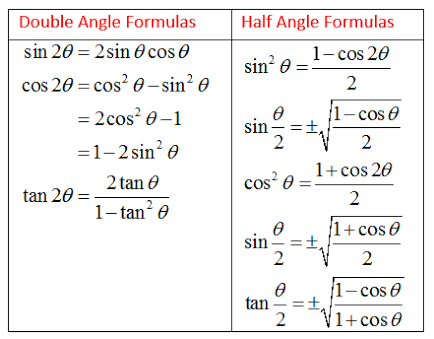


Double Angle Identities Trigonometry Socratic
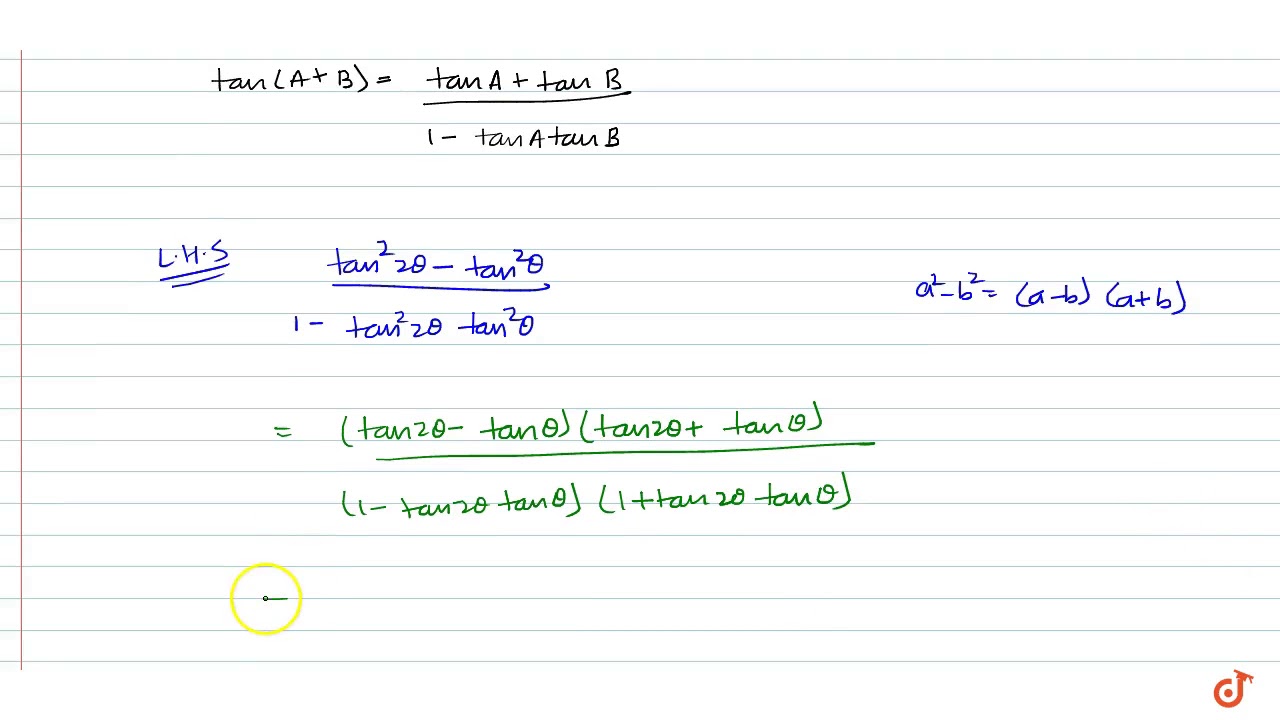


Prove That Tan 2 2theta Tan 2 Theta 1 Tan 2 2theta Tan 2 Theta Tan 3theta Tan Theta Youtube
Prove that c o sec 2 θ (1 tan 2 θ) cot θ = tan θ View solution In a right angle triangle ABC , right angle is at B , if tan A = 3 then find the value ofIntroduction to Tan double angle formula let's look at trigonometric formulae also called as the double angle formulae having double angles Derive Double Angle Formulae for Tan 2 Theta \(Tan 2x =\frac{2tan x}{1tan^{2}x} \) let's recall the addition formulaTrigonometry Solve for ?



The Reciprocal Trigonometric Functions Problem 3 Trigonometry Video By Brightstorm



Art Of Problem Solving
Question if cos theta= 3x, then tan^2 theta=?If `tan\ theta/2=(cosec thetasin theta),` then `tan^2\ theta/2` may be equal to (A) `2sqrt(5)` (B) `(94sqrt(5))(2sqrt(5))` `2sqrt(5)` (D) `(94sqrtLet's do some examples simplifying trigonometric expressions so let's say that I have 1 minus sine squared theta and this whole thing times cosine cosine squared theta so how could I simplify this well the one thing that we do know this is the most fundamental trig identity this comes straight out of the unit circle is that cosine squared theta plus sine squared theta is equal to 1 and then if



If Tan Theta Sec Theta 2 Then What Is Tan Theta Is Equal To Quora



Same As In Fig 3 But With Values Of 3 And Tan 2 8 0 Equal To Those Of Download Scientific Diagram
This article uses Greek letters such as alpha (α), beta (β), gamma (γ), and theta (θ) to represent anglesSeveral different units of angle measure are widely used, including degree, radian, and gradian () 1 full circle () = 360 degree = 2 π radian = 400 gonIf not specifically annotated by (°) for degree or for gradian, all values for angles in this article are assumed to be given inAnswer by jsmallt9(3758) (Show Source) You can put this solution on YOUR website!Tan (theta)=2 tan (θ) = 2 tan (θ) = 2 Take the inverse tangent of both sides of the equation to extract θ θ from inside the tangent


Prove That 1 Tan 2 8 1 Cot 2 8 1 Tan 8 Cot 8 2 Tan 2 8 Sarthaks Econnect Largest Online Education Community


Tan Theta Equals 0 General Solution Of The Equation Tan 8 0 Tan 8 0
Tan (theta) = 2 this means that opposite divided by adjacent is equal to 2 this can occur if opposite = 2 and adjacent = 1 this would make hypotenuse equal to sqrt (2^2 1^2) = sqrt (5)$$\int\frac{\sec\theta}{\tan^2\theta}d \theta$$ I know by head that the answer is $$\csc\thetaC$$ However, I could not figure out how to do it manually I've first tried to transform the integral to $$\int\csc\theta\cot\theta$$ and thenT a n 2 θ − s e c 2 θ is equal to A 1 B1 C 0 D c o t 2 θ Easy Answer Correct option is B1 As we know that, sin 2 θ cos 2 θ = 1 tan 2



Solved Write The Value Of 4 Tan2 8 4cos2 8 Self Study 365



Prove That Sin Theta Minus Cos Theta 1 Divided By Sin Theta Cos Theta Minus 1 Is Equal To 1 Sec Msrblog
Jul 15, 18 · How do you apply the fundamental identities to values of #theta# and show that they are true?2sec^2 theta tan ^2 theta tan^4 theta = sec ^4 theta 1 Factor the left side of the identity Apply a Pythagorean identity to rewrite the above expression entirely in terms of sec theta and simplify each factor Do not multiply The above expression is shown to be equal to the right side of the identity after applying which of the following?Nov 01, 17 · Stack Exchange network consists of 176 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers Visit Stack Exchange



Prove That Tan 3 Theta 1 Tan 2theta Cot 3 Theta 1 Cot 2 Theta



14 2 Trigonometric Identities
And the above approximation follows when tan X is replaced by X Motion of a pendulum The secondorder cosine approximation is especially useful in calculating the potential energy of a pendulum, which can then be applied with a Lagrangian to find the indirect (energy) equation of motion When calculating the period of a simple pendulum, the smallangle approximation forThere's probably more than one way to solve this Perhaps the easiest is based on recognizing that cos and tan are both connected to sec andFree online tangent calculator tan(x) calculator This website uses cookies to improve your experience, analyze traffic and display ads
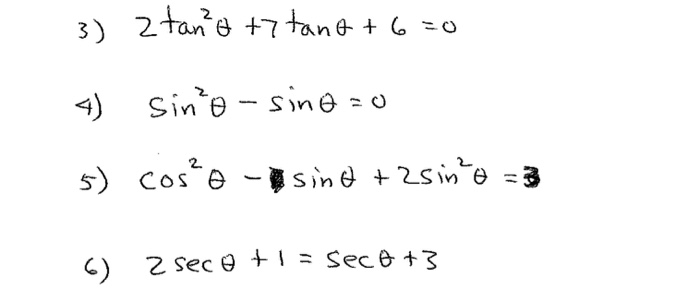


Solved 2 Tan 2 Theta 7 Tan Theta 6 0 Sin 2 Theta Chegg Com
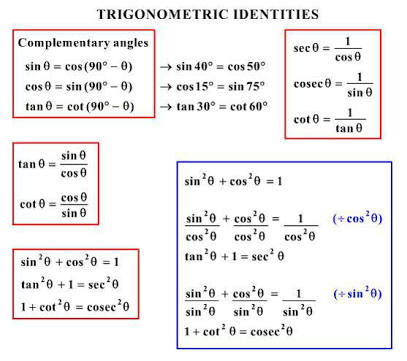


How Do You Simplify The Expression Sin 2theta Cos 2theta Cos 2theta Socratic
Well, for this, we start by using the arctan function, which is the inverse of the tangent function and finds a value math\theta/math such that math\tan(\theta) = 2/math We can compute the value, but this isIf ` sin theta = cos theta` ,then the value of `2 tan^(2) theta sin^(2) theta 1` is equal to



If Tan 3 Theta Cot 3 Theta 52 Then The Value Of Tan 2 Theta Cot 2 Theta Is Equal To Youtube



Prove That 1 Tan 28 1 Cot 28 1 Tan8 1 Cot8 2 Tan 28


If Tan 2theta 1 2tan 2a Then Prove That 2sin 2theta 1 Sin 2a Mathematics Topperlearning Com 47



Prove That 1 1 Tan 2 Theta 1 1 Cot 2 Theta 1
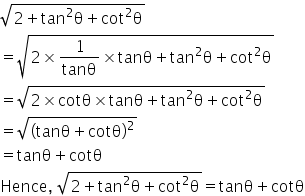


Prove That Radic 2 Tan2 Theta Cot2 Theta Tan Theta Cot Theta Mathematics Topperlearning Com Ttevdk55



Tangent Half Angle Formula Wikipedia
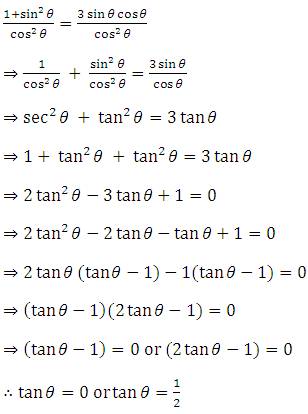


1 Sin2 Theta 3 X Sin Theta X Cos Theta Introduction To Trigonometry Maths Class 10
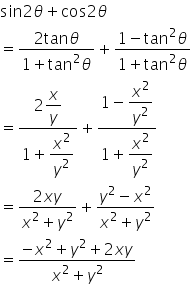


If Tan Theta X Y Then Find Sin 2 Theta Cos 2 Theta Mathematics Topperlearning Com 3co7touu



Prove That A Sin 2 Theta 1 Cos 2 Theta Tan Theta B 1 Sin 2 Theta 2theta Youtube
x-1=sec(squared)x.jpg)


10 Identity Tan Squared X 1 Sec Squared X Trigonometry Educator Com



Sec Theta Minus Tan Theta By Sin Theta Tan Theta Equal To 1 2 Tan Squared Theta Minus Maths Introduction To Trigonometry Meritnation Com
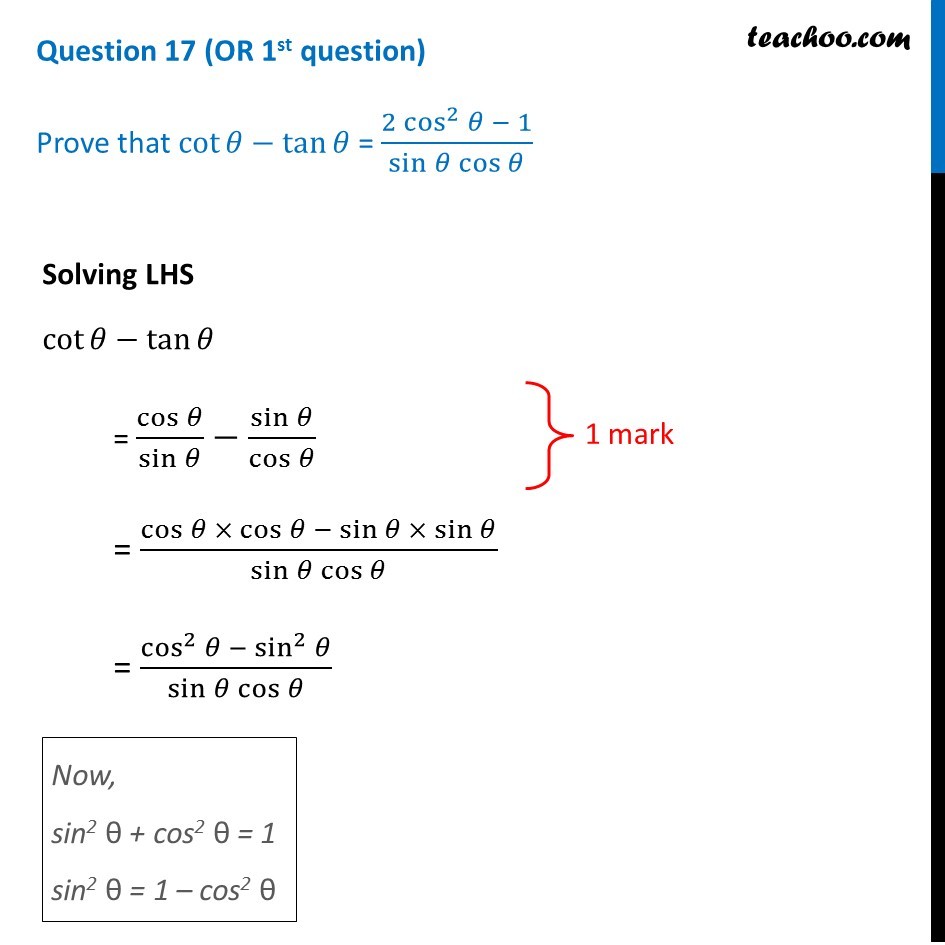


Prove That Cot 8 Tan 8 2 Cos 2 8 1 Sin 8 Cos 8


What Is The Formula Of Tan2x Quora


What Is The Formula Of Tan2x Quora



1 Tan 2theta Cot Theta Cosec 2 Theta Tan Theta Brainly In



Tangent Half Angle Formula Wikipedia



If Tan Theta Cottheta 6 Then The Value Of Tan 2theta Cot 2theta Is
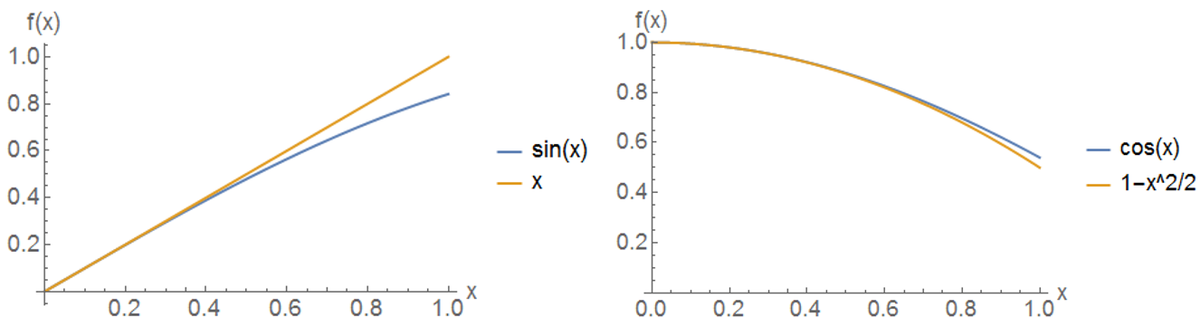


Small Angle Approximation Brilliant Math Science Wiki


If Tan Theta Cot Theta 2 Then What Is The Value Of Tan Squared Theta Cot Squared Theta Equal To Quora



14 2 Trigonometric Identities


Prove That Math Tan 2 Theta 1 Sec 2 Theta Math Quora



Prove That One Plus Tan Squared Theta Plus One Plus One By Tan Squared Theta Is Equal To One By Brainly In



Prove That Sqrt Sec 2 Theta Cosec 2 Theta Tan Theta Cot Theta Youtube



Solved Prove The Following Identities Tan 2theta 2 Tan Chegg Com



Trigonometric Identities Ppt Download
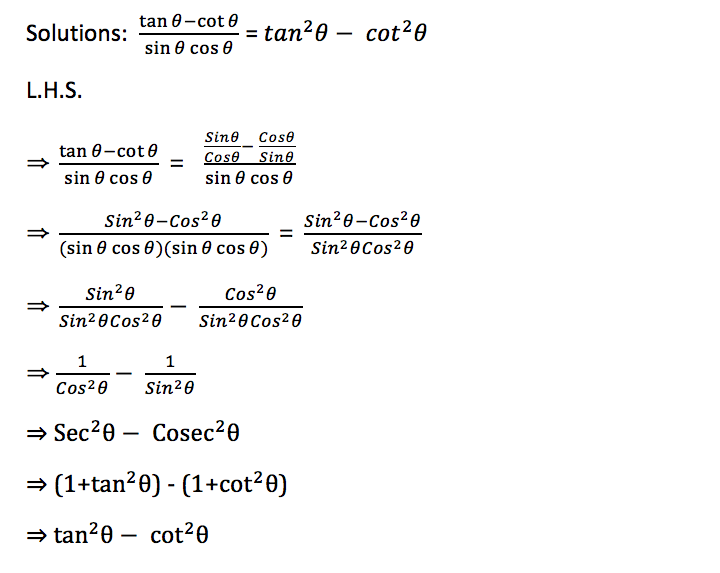


Tan Theta Cot Theta Sin Theta Cos Theta Tan 2 Theta Cot 2 Theta Class Of Achievers



3 4sin 2 Theta Cos 2 Theta Is Equal To 3 Tan 2 Theta Maths Some Applications Of Trigonometry Meritnation Com


Prove That A X B Y 1 Introduction To Trigonometry Maths Class 10
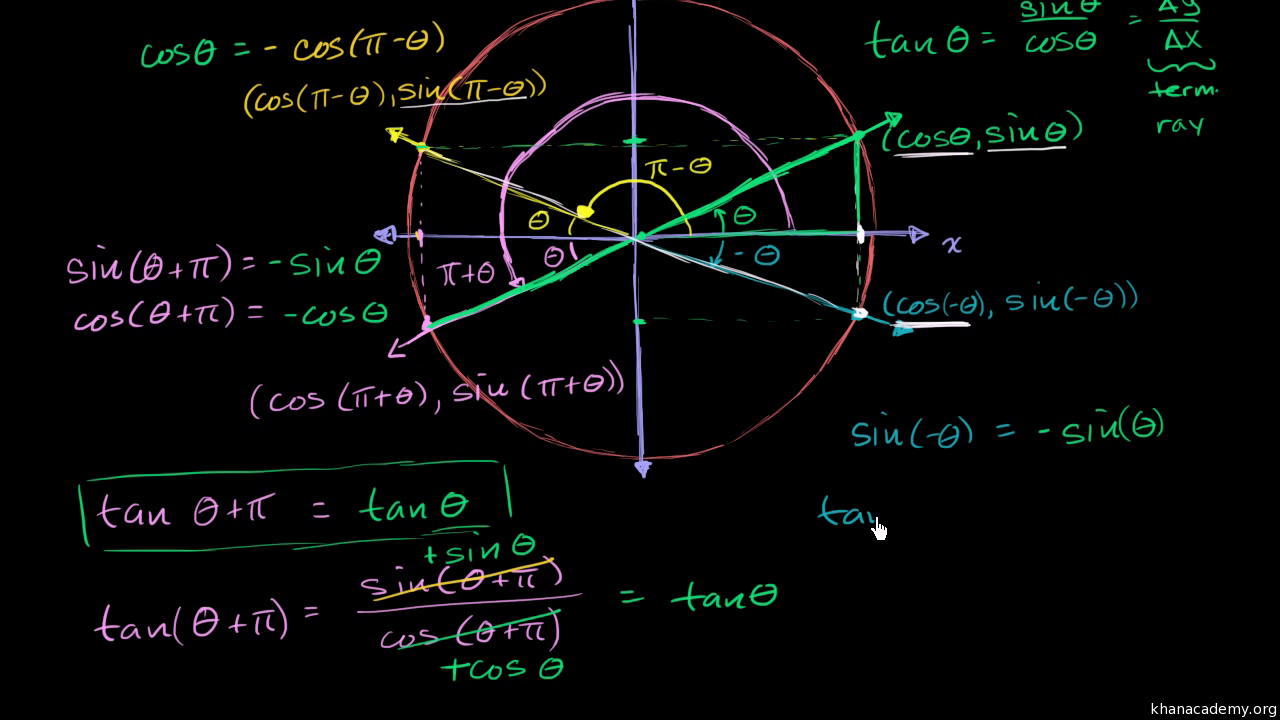


Tangent Identities Symmetry Video Khan Academy



Prove That 2 Sin Theta Sin 2theta 2 Sin Theta Sin 2theta Tan 2 Theta 2 Youtube



If Theta 60 Then 1 Tan 2 Theta 2tan Theta Is Equal To
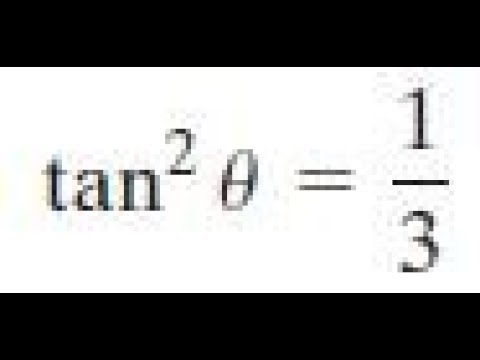


Tan 2 Theta 1 3 Solve For Interval 0 Less Theta Less 2pi Youtube


If M Cos8 Sin8 And N Cos8 Sin8 Then Show That M N N M 2 1 Tan 2 8 Sarthaks Econnect Largest Online Education Community



If A 2 Sec 2 Theta B 2 Tan 2 Theta C 2 Prove That Sin Theta Plusmn Mathematics Topperlearning Com Q5azu9oo



14 2 Trigonometric Identities
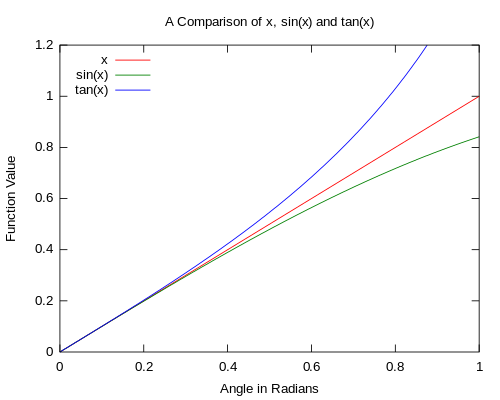


Small Angle Approximation Wikipedia



Prove That Cot 8 Tan 8 2 Cos 2 8 1 Sin 8 Cos 8



Two Solutions Of Tan 2 8 12 0 45 Corresponding To H ǫ Equals To Download Scientific Diagram



If Sin 8 Cos 8 Then Find The Value Of 2 Tan 8 Cos 2 8 Teachoo



Prove That Tan 2 Theta Cot 2theta 2 Sec 2theta Cosec 2theta Brainly In



Summary Of Trigonometric Identities



Solved 1 Tan Squared Theta Into 1 Cot Square Theta Is Equals To 1 By Sin Squared Theta Minus Sin Brainly In


The Trigonometric Ratios Of Angl
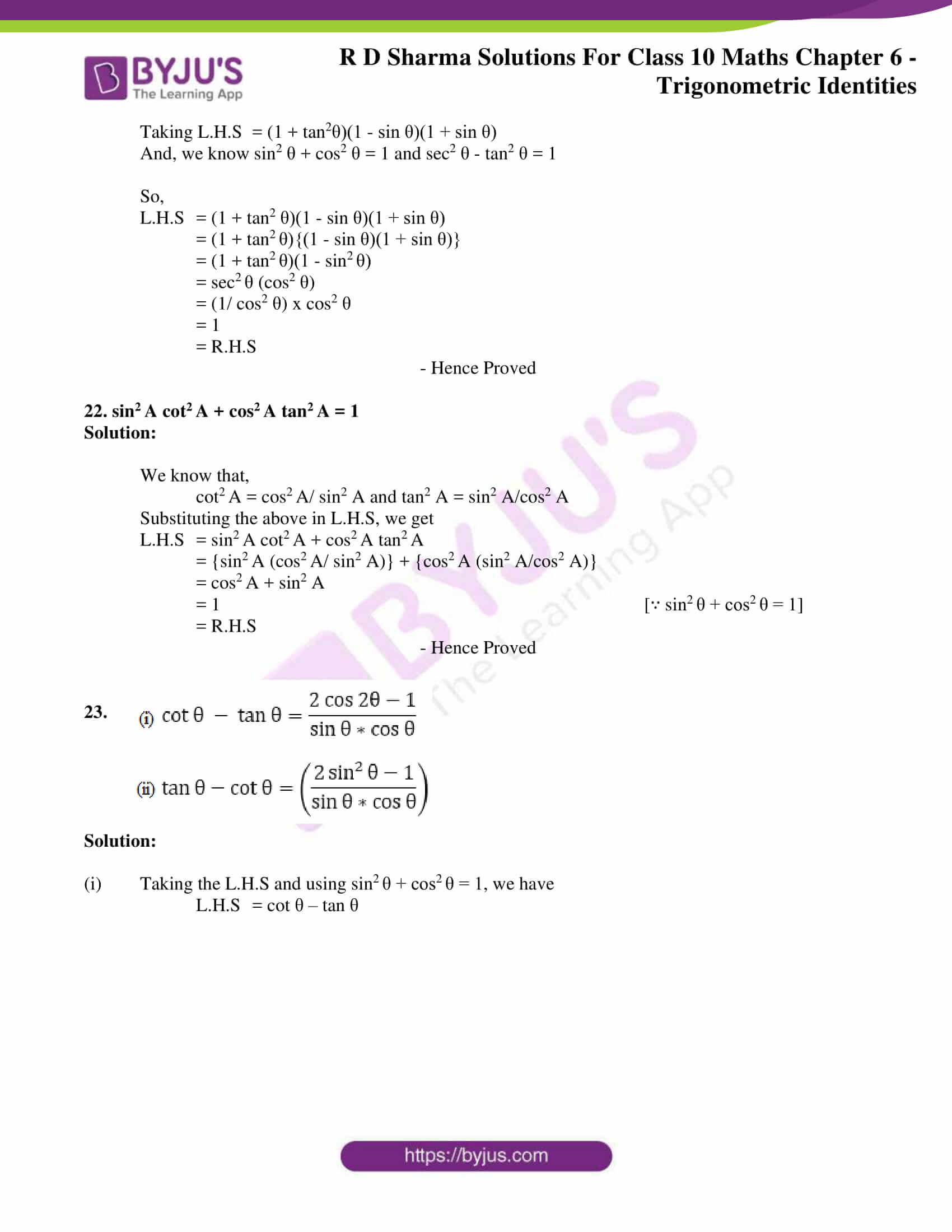


Rd Sharma Solutions For Class 10 Chapter 6 Trigonometric Identities Exercise 6 1 Get Pdf
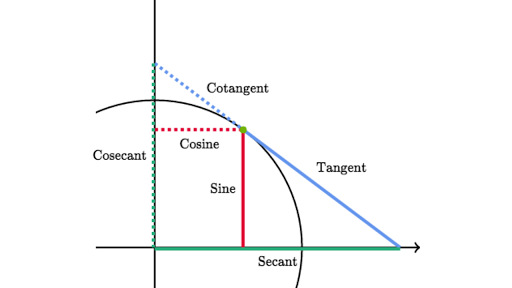


Trig Identity Reference Article Khan Academy


Prove P2 1 P2 1 Sin Theta Introduction To Trigonometry Maths Class 10
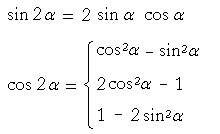


Trigonometric Identities Topics In Trigonometry



Solved Which Expression Is Equivalent To Tan 2 Theta Si Chegg Com


The Trigonometric Ratios Of Angl


If 15 Tan 28 4sec 28 23 Then Find The Value Of Sec 8 Cosec 8 2 Sin 28 Sarthaks Econnect Largest Online Education Community



Numbers Of Solutions Of The Equation Tan 2theta Tan 6theta In



Trigonometric Identities



If Tan Theta 1 3 Then Evaluate Cosec 2 Theta Sec 2 Theta Cosec 2 Theta Sec 2 Edurev Class 10 Question


If Tan 8 1 Tan 8 2 What Is The Values Of Tan 8 1 Tan 8 Quora
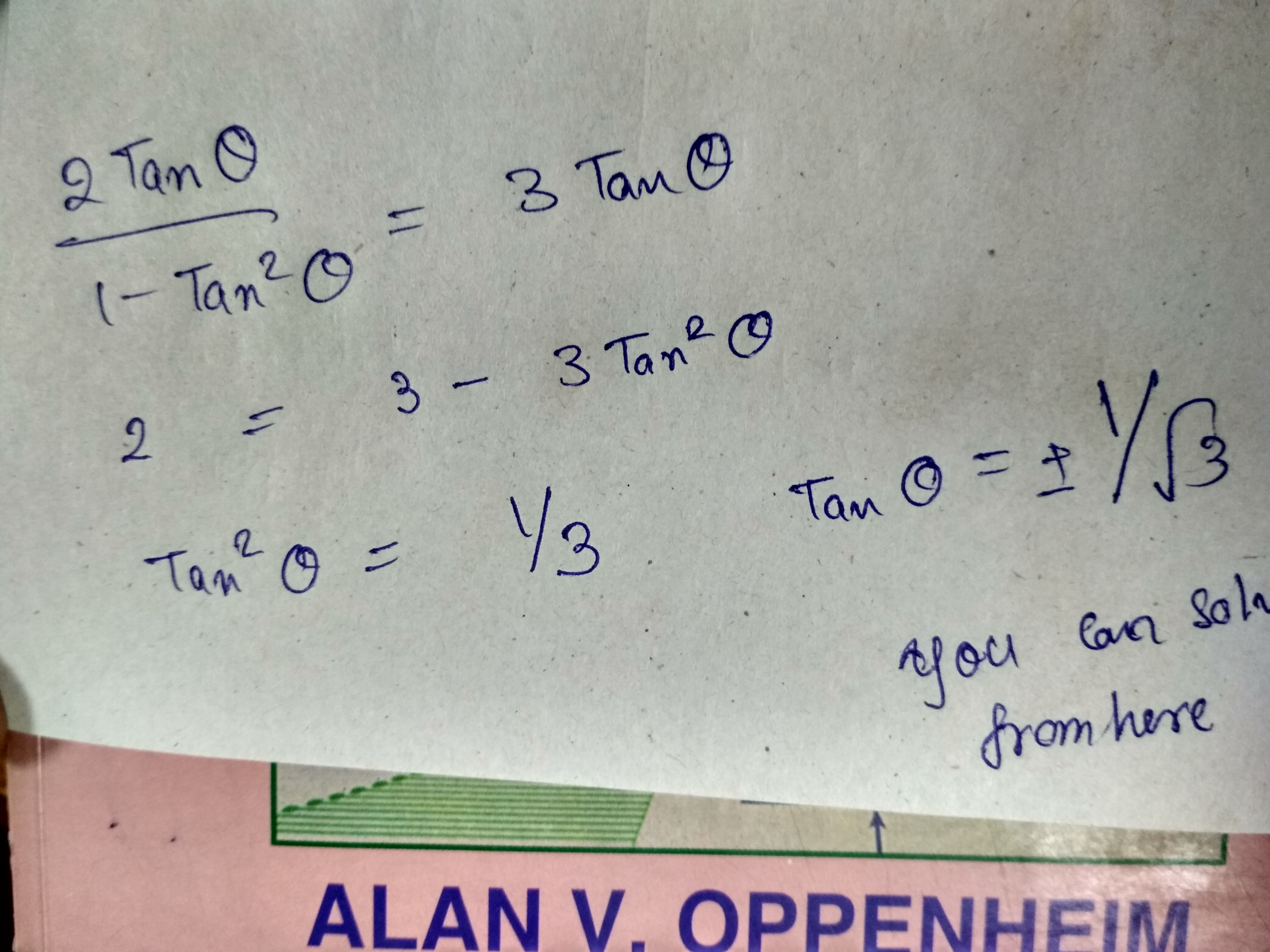


Tan 2theta Equal To 3 Tan Theta Solve To Get Theta Askiitians



Ganitaacademy If 2 Sin Theta 2 Costheta 1 Then Prove That 2 Sin Theta Cos Theta 2 Facebook



Find The Value Of Theta If Root 3 Tan 2 Theta 3 0 Maths Introduction To Trigonometry Meritnation Com



Tangent Half Angle Formula Wikipedia


Solution Prove That Sin Theta Times Cos Theta Divided By Cos 2 Theta Sin 2 Theta Tan Theta Divided By 1 Tan Theta Show Step By Step How To Change The Left To Equal The Right Side Of E
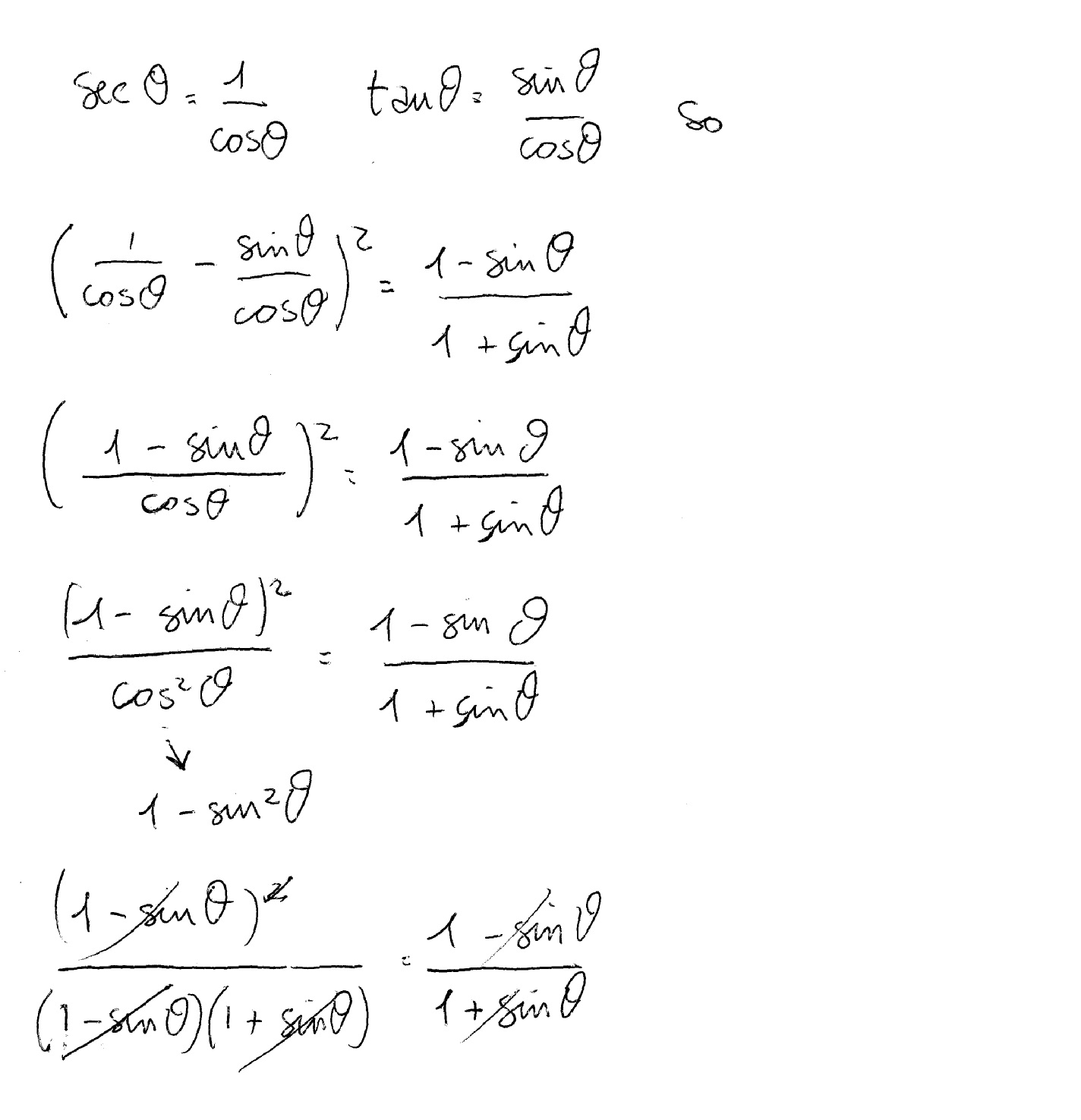


How Do You Prove The Identity Sectheta Tantheta 2 1 Sintheta 1 Sintheta Socratic



How Do You Prove Tan 2x Secx 1 1 Secx Socratic


Cbse 10 Math Cbse Introduction To Trigonometry Ncert Solutions
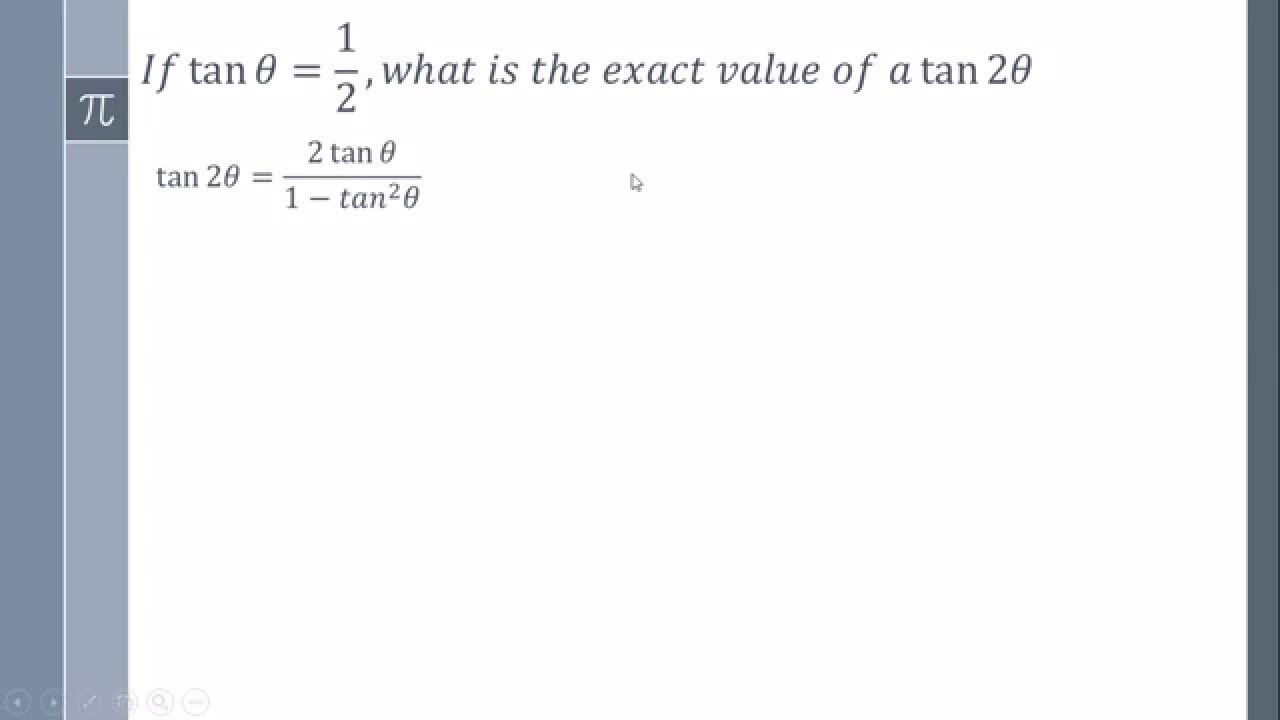


If Tan Of Theta Is 1 2 What Is The Exact Value Of Tan 2 Theta Youtube
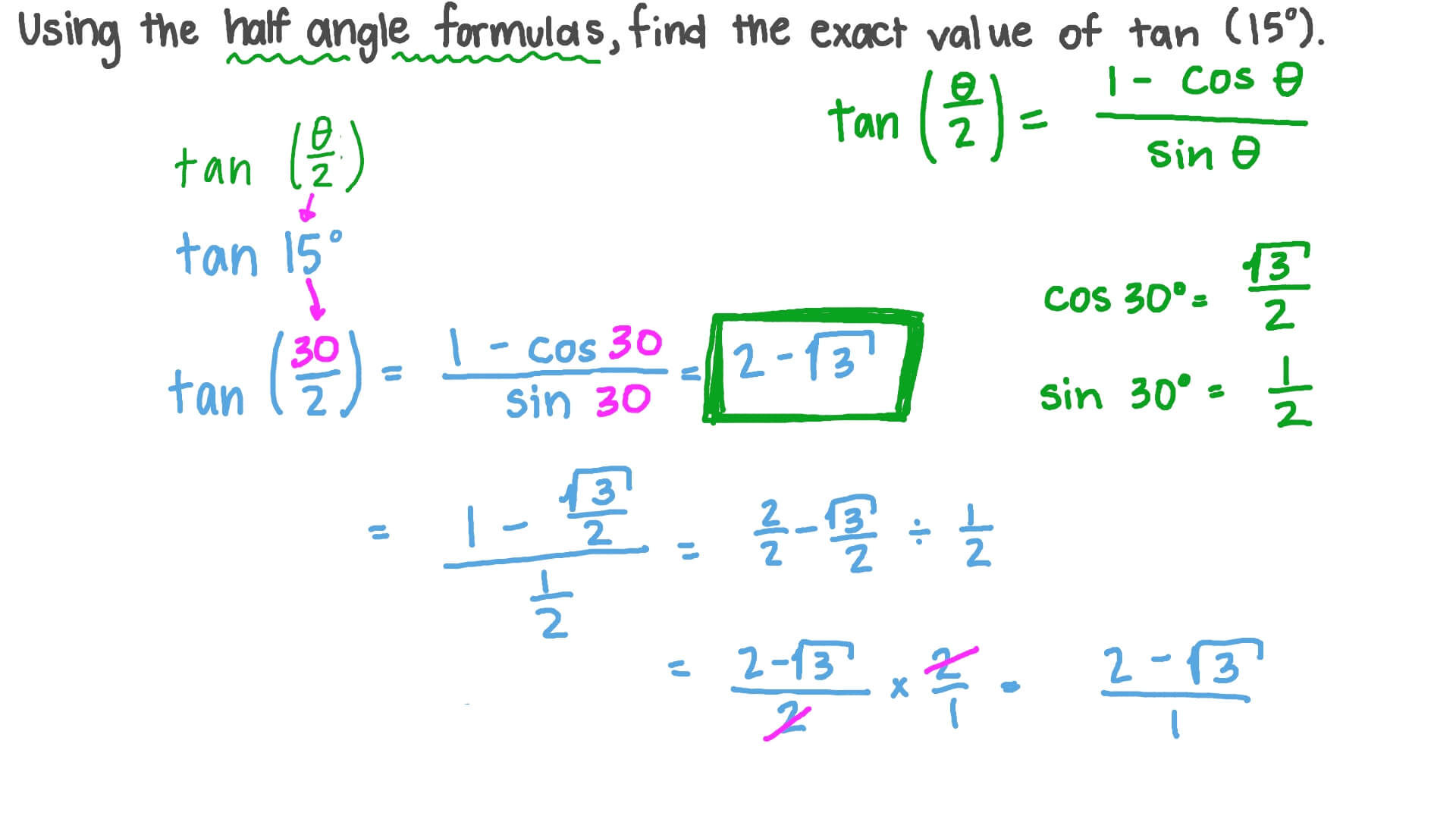


Question Video Using The Half Angle Formulas To Find The Exact Value Of A Trigonometric Expression Nagwa



If Tan Theta Cot Theta Is Equal To 7 Find The Value Of Tan Squared Theta Cos Squared Maths Introduction To Trigonometry Meritnation Com



If Sin Theta Cos Theta Then The Value Of 2 Tan 2 Theta Sin 2 Theta 1 Is Equal To Youtube



Cos Squared Theta Minus Sin Square Theta Equal To 1 Minus 10 Squared Theta By 1 10 Squared Theta Prove The Identity Class Of Achievers



1 1 Tan 2 Theta 1 1 Cot 2 Theta 1 Sin 2 Theta Sin 4 Theta Brainly In



Tan 2 Theta Cos 2 Theta 1 Cos 2 Theta Brainly In
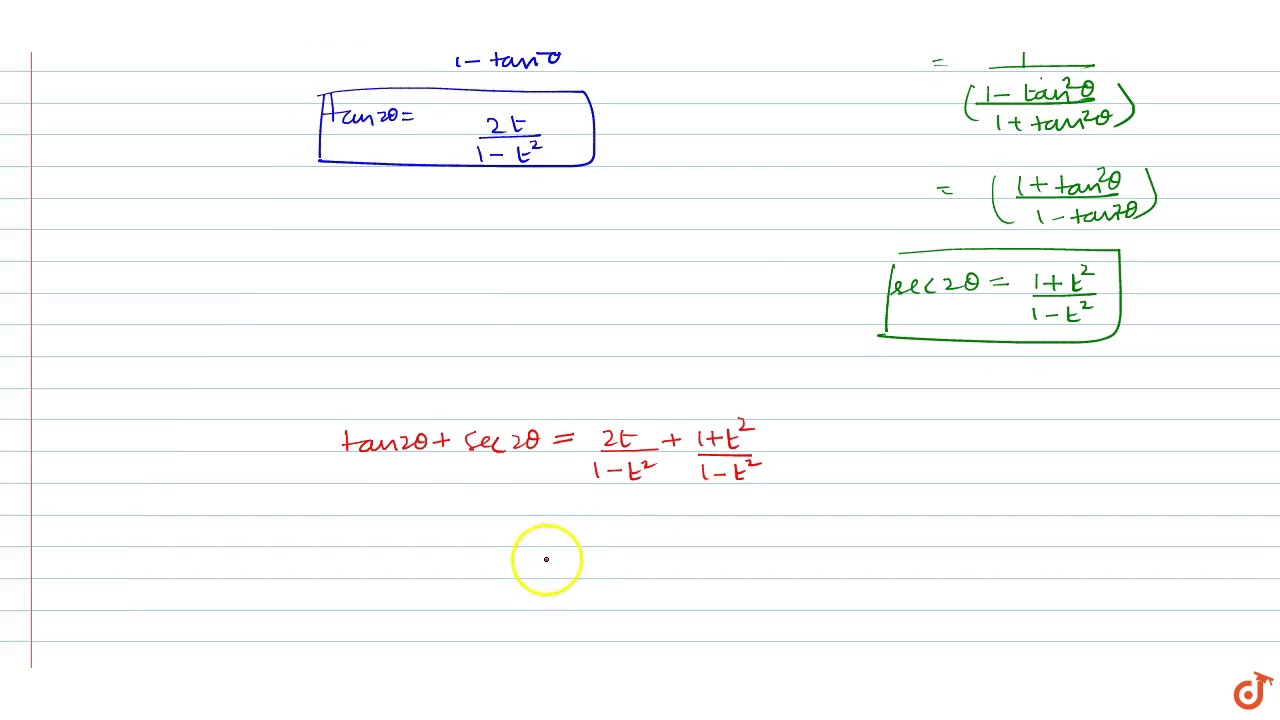


If Tan Theta T Then Tan 2 Theta Sec 2 Theta Youtube
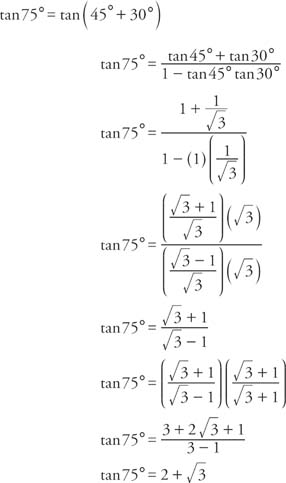


Tangent Identities
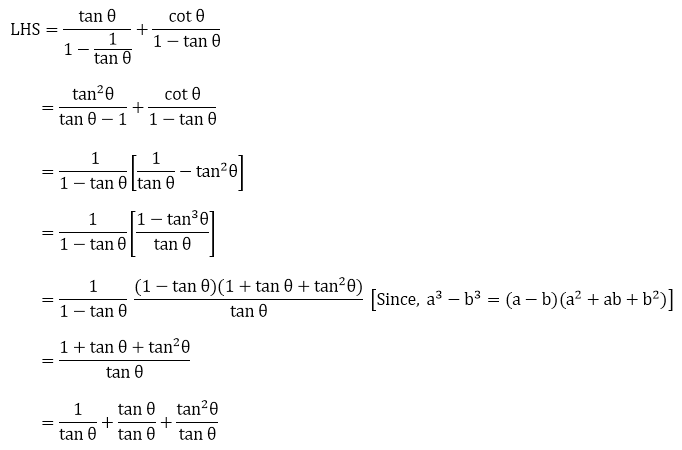


Rd Sharma Solutions For Class 10 Chapter 6 Trigonometric Identities Exercise 6 1 Get Pdf



The Derivative Of Tan 2x Derivativeit


The Trigonometric Ratios Of Angl


Content Graphing The Trigonometric Functions



Tan 4 Theta 4 Tan Theta 1 Tan 2 Theta 1 6 Tan 2 Theta Tan 4 Theta Maths Trigonometric Functions Meritnation Com



Tan 2theta Sin 2theta Tan 2theta Sin 2theta


How To Solve Tan Theta 2 Quora



0 件のコメント:
コメントを投稿